Mohr's Circle

Mohr's Circle for 2D

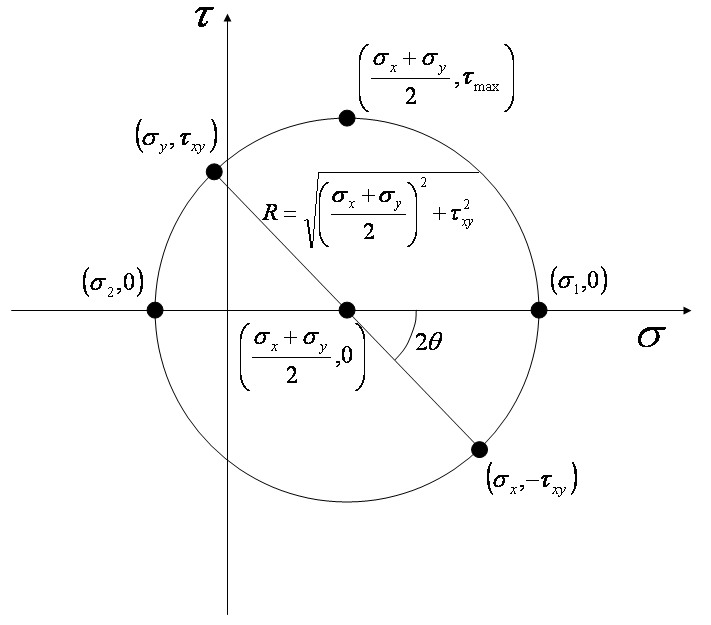
Mohr's circle is a tool for analyzing element stress. The drawing below shows mohr's circle and some of the relavent points of interest.

Given a state of stress, $(\sigma _{x},\sigma _{y},\tau _{xy})$, we can calculate the principle stresses,

$\sigma _{1}={\frac {1}{2}}\left(\sigma _{x}+\sigma _{y}\right)+{\frac {1}{2}}{\sqrt {\left(\sigma _{x}-\sigma _{y}\right)^{2}+4\tau _{xy}^{2}}}$

$\sigma _{2}={\frac {1}{2}}\left(\sigma _{x}+\sigma _{y}\right)-{\frac {1}{2}}{\sqrt {\left(\sigma _{x}-\sigma _{y}\right)^{2}+4\tau _{xy}^{2}}}$