Difference between revisions of "Brushed DC Motor Theory"
m (→Motor Physics) |
|||
| Line 106: | Line 106: | ||
==References== |
==References== |
||
* Carl R. Nave, "DC Motors," http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/motdc.html |
* Carl R. Nave, "DC Motors," http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/motdc.html |
||
* Maxon DC Motor ([[media:Maxon Motor Guide.pdf|pdf]]) |
|||
Revision as of 13:12, 19 July 2006
Introduction
The specific type of motor we are addressing is the permanent magnet brushed DC motor (PMDC). These motors have two terminals. Applying a voltage across the terminals results in a proportional speed of the output shaft.
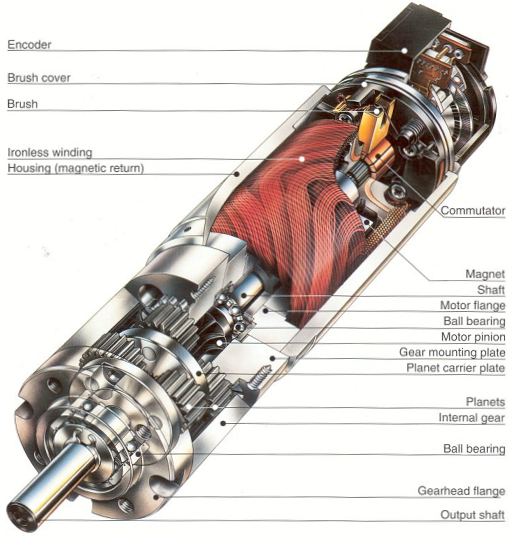
There are two pieces to the motor: 1) stator and 2) rotor. The stator includes the housing, windings and brushes. The rotor consists of the output shaft, windings and commutators. The image below shows a cut-away view of a Maxon motor. Note this picture has a gearbox and encoder attached to the motor.
Motor Physics
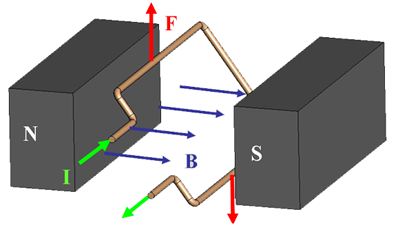
The forces inside a motor that cause the rotor to rotate are called Lorentz Forces. If an electron is moving through an electric field, it experiences a force that is perpendicular to both the magnetic field and the direction it's moving. If we have a wire instead of a single electron, the wire experiences a force equal to
You can easily find the direction of this force using the Right Hand Rule. The Right Hand Rule states that if you point your right hand fingers along the direction of current, , and curl them towards the direction of the magnetic flux, , the direction of force is along the thumb. See the picture below.
Now, imagine this single wire is replaced with a loop of wire. Between the magnets poles, this looks like two wires with current flowing in opposite directions. The forces on the wires cause the loop to rotate with forces as shown.
This coil is attached to the rotor and rotates. As it does so, the magnitude and direction of the force on the wires remains constant. However, the resultant torque varies with the angle. Look at the picture below. When the coil starts, there is maximum torque. As the coil moves, the moment arm is reduced and the torque decreases. Finally, when the coil is vertical (in the picture), there is no torque.
To keep a (almost) constant torque on the rotor, there are two things that happen. First, the current through the coil is reversed every half turn. So instead of an alternating torque like the one in the first figure below, the torque is always in the same direction. Also, additional coils can be used. When these coils are offset at different angles around the motor, the resultant torque becomes the sum of the colored torque curves in the figure below. The resultant torque is alway greater than zero, but is not constant. This variation is called torque ripple.
The process of switching current direction is called commutation. To switch the direction of curent, brushed DC motors use brushes and commutators. Commutation can also be done electronically (see Brushless DC Motors). The following diagram shows how brushes and commutators work.
Equations
So, the torque is proportional to the current through the windings,
,
where is the torque, is the current, and is a constant. The wire coils have both a resistance, , and an inductance, . When the motor is turning, the current is switching, causing a voltage,
This voltage is known as the back-emf(electromotive force), . If the angular velocuty of the motor is , then
,
like a generator. This voltage, , is working against the voltage we apply across the terminals, and so,
,
where
which implies
.
The maximum or stall torque is the torque at which or
,
and the stall or starting current,
The no load speed,
,
is the maximum speed the motor can run. Given a constant voltage, the motor will settle at a constant speed, just like a terminal velocity. If we plot
,
we can get a linear speed-torque curve. This line is the dashed line shown in the dc motor speed-torque graph for a Maxon brushed DC motor shown below:
Units
Here are the different units for the torque, current and voltage
| Parameter | Symbol | Common Units |
|---|---|---|
| Torque | Nm (=kgm/s^2*m), kgfm(=9.8 times Nm), gfcm, mNm, etc | |
| Current | Amperes(Amps), mA | |
| Voltage | Volts | |
| Mechanical Power | 1 Nm/sec = 1 watt | |
| Electrical Power | 1 volt*amp = 1 watt |
References
- Carl R. Nave, "DC Motors," http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/motdc.html
- Maxon DC Motor (pdf)